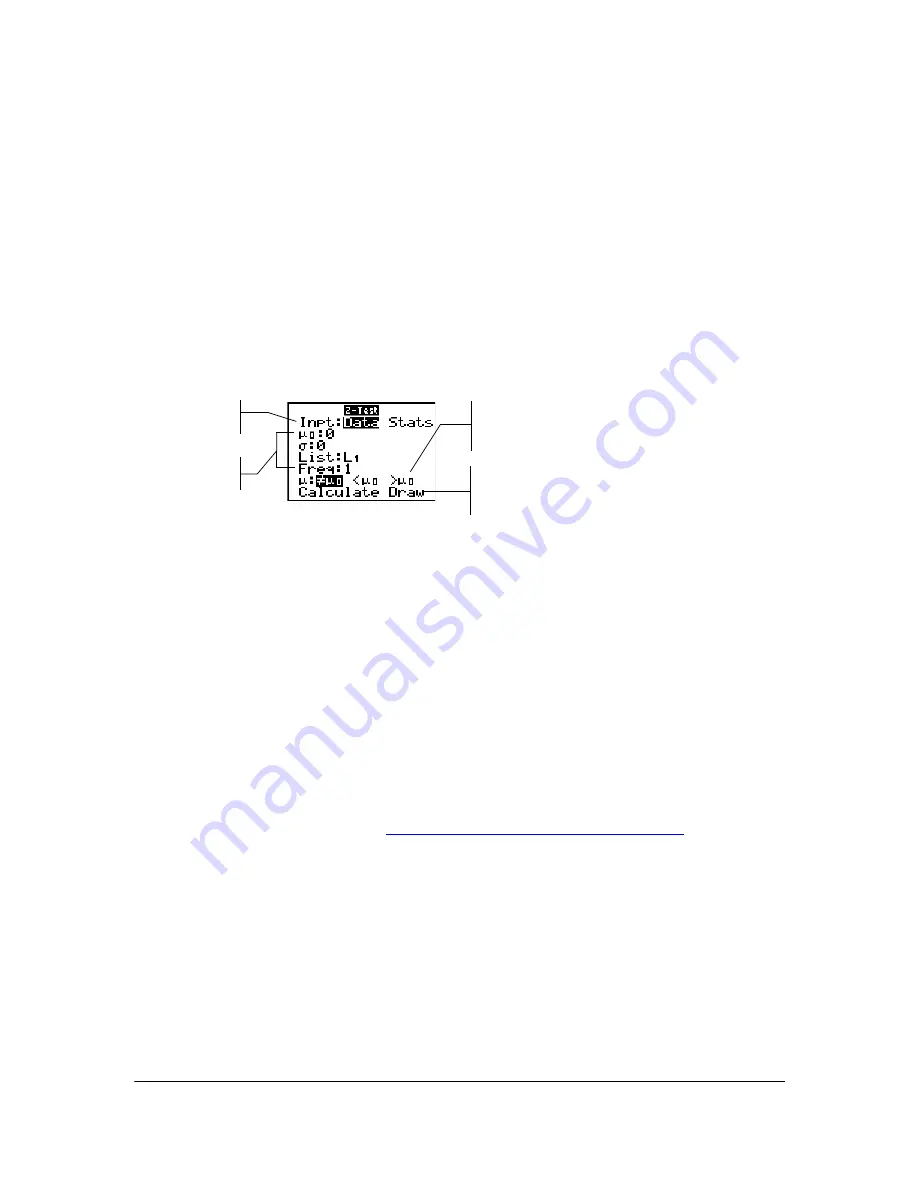
Chapter 13: Inferential Statistics and Distributions
219
1. Select a hypothesis test or confidence interval from the
STAT TESTS
menu. The appropriate
editor is displayed.
2. Select
Data
or
Stats
input, if the selection is available. The appropriate editor is displayed.
3. Enter real numbers, list names, or expressions for each argument in the editor.
4. Select the alternative hypothesis (
Ā
,
<
, or
>
) against which to test, if the selection is available.
5. Select
No
or
Yes
for the
Pooled
option, if the selection is available.
6. Select
Calculate
or
Draw
(when
Draw
is available) to execute the instruction.
•
When you select
Calculate
, the results are displayed on the home screen.
•
When you select
Draw
, the results are displayed in a graph.
Select Data or
Stats input
Select an
alternative
hypothesis
Enter values
for arguments
Select
Calculate or
Draw output
This chapter describes the selections in the above steps for each hypothesis test and confidence
interval instruction.
Selecting Data or Stats
Most inferential stat editors prompt you to select one of two types of input. (
1-PropZInt
and
2-PropZTest
,
1-PropZInt
and
2-PropZInt
,
c
2
-Test
,
c
2
GOF-Test
,
LinRegTInt
,
and
LinRegTTest
do not.)
•
Select
Data
to enter the data lists as input.
•
Select
Stats
to enter summary statistics, such as
v
,
Sx
, and
n
, as input.
To select
Data
or
Stats
, move the cursor to either
Data
or
Stats
, and then press
Í
.
Entering the Values for Arguments
Inferential stat editors require a value for every argument. If you do not know what a particular
argument symbol represents, see the
Inferential Statistics Input Descriptions tables
When you enter values in any inferential stat editor, the TI-84 Plus stores them in memory so that
you can run many tests or intervals without having to reenter every value.
Selecting an Alternative Hypothesis (
ă
< >)
Most of the inferential stat editors for the hypothesis tests prompt you to select one of three
alternative hypotheses.
•
The first is a
ƒ
alternative hypothesis, such as
mƒm
0 for the
Z-Test
.
•
The second is a
<
alternative hypothesis, such as
m
1<
m
2 for the
2-SampTTest
.






























