3
3.2 Formulas and Calculation of Derived Quantities
44
Power Meter SICAM P 7KG7750/55, Manual
E50417-B1076-C340-A6, Edition 09.2013
3.2
Formulas and Calculation of Derived Quantities
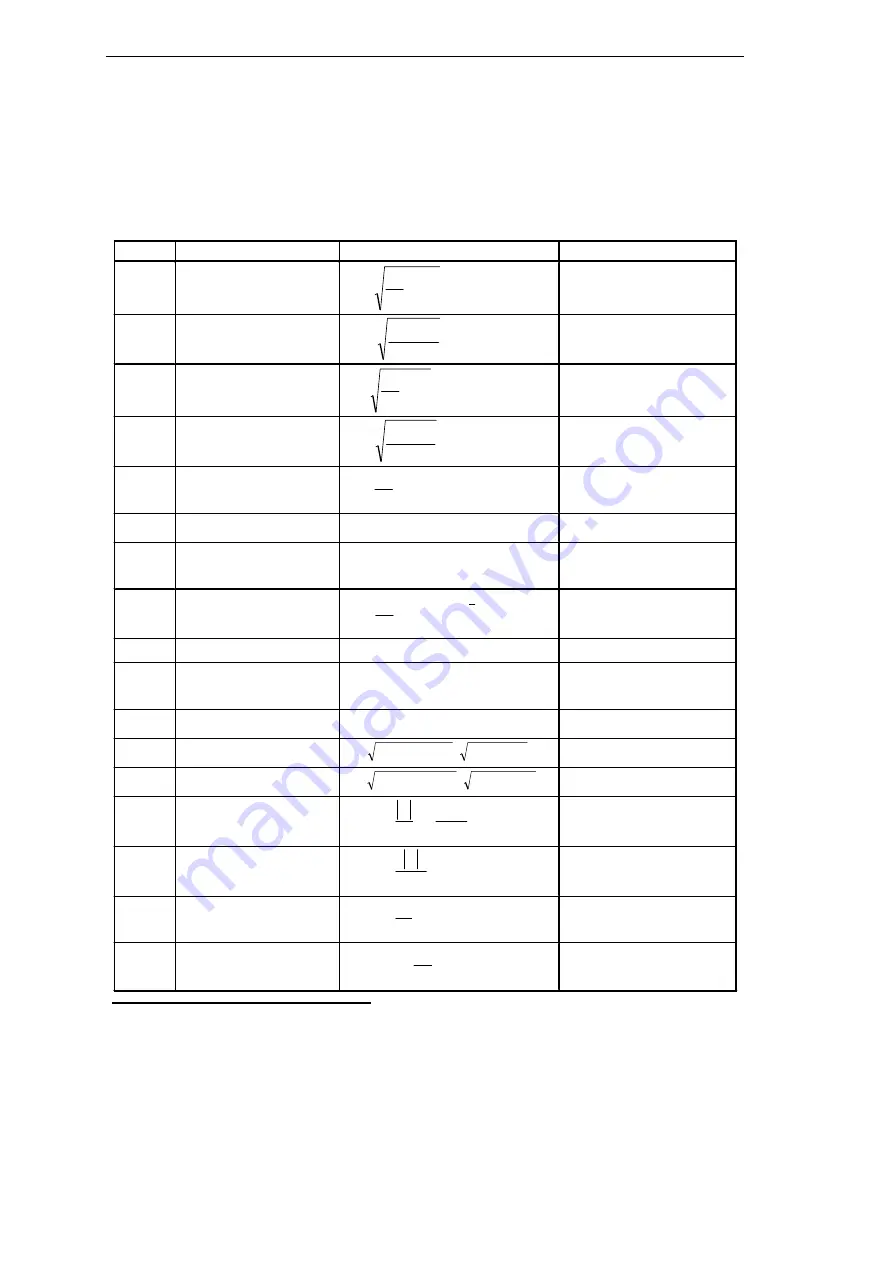
3.2.1
Calculation of Derived Quantities
Line Derived
Quantity
Formula
Note
1
RMS value voltage,
distorted waveform included
∑
=
=
64
1
2
64
1
ν
ν
u
V
2
RMS value voltage,
fundamental component U
1
only
2
2
2
1
b
a
V
+
=
From the Fourier coefficients a
and b of the fundamental
component
3
RMS value current,
distorted waveform included
∑
=
=
64
1
2
64
1
ν
ν
i
I
4
RMS value voltage,
fundamental component I
1
only
2
2
2
1
b
a
I
+
=
From the Fourier coefficients a
and b of the fundamental
component
5
Active power P
Std
∑
=
=
64
1
64
1
ν
ν
ν
i
v
P
From sample values
6
Active power P
Four
1
1
1
1
Ib
Vb
Ia
Va
P
+
=
From the Fourier coefficients
of the fundamental component
7
Active power P
DIN
)
(
21
1
n
n
n
n
n
Ib
Vb
Ia
Va
P
+
=
∑
=
From the Fourier coefficients
of the fundamental component
and from the harmonics.
8
Reactive
power
Q
Std
∑
=
−
⋅
=
64
1
2
1
64
1
ν
π
ν
ν
j
e
i
v
Q
Standard up to now, additional
fault for distortions
1
9
Reactive
power
Q
Four
1
1
1
1
Ia
Vb
Ib
Va
Q
+
=
10 Reactive
power
Q
DIN
)
(
21
1
n
n
n
n
n
tot
Ia
Vb
Ib
Va
Q
+
=
∑
=
From the Fourier coefficients
of the fundamental component
11
Apparent power S
Std
3
3
2
2
1
1
I
V
I
V
I
V
S
N
N
N
⋅
+
⋅
+
⋅
=
From the RMS values
according to line 1 and 3
12
Apparent power S
Four
2
3
2
2
2
1
2
3
2
2
2
1
I
I
I
V
V
V
S
N
N
N
+
+
⋅
+
+
=
From the RMS values
according to line 1 and 3
13
Apparent power S
DIN
2
3
2
2
2
1
2
3
2
2
2
1
I
I
I
V
V
V
S
N
N
N
+
+
⋅
+
+
=
From the RMS values
according to line 2 and 4
14 Power
factor
S
P
=
ϕ
cos
or
DIN
S
P
1
No sign!
15
Power factor DIN
DIN
S
P
=
ϕ
cos
No sign!
16 Power factor cos
ϕ
1
1
cos
S
P
=
ϕ
Four quadrants according to
note 4
17 Phase
Angle
1
1
arctan
P
Q
=
ϕ
From the fundamental
component only!
1
According to classic measuring devices (electrodynamic power meter)






























