Texas Instruments Titanium TI-89, User Manual

Page: 733 / 977

Activities 730
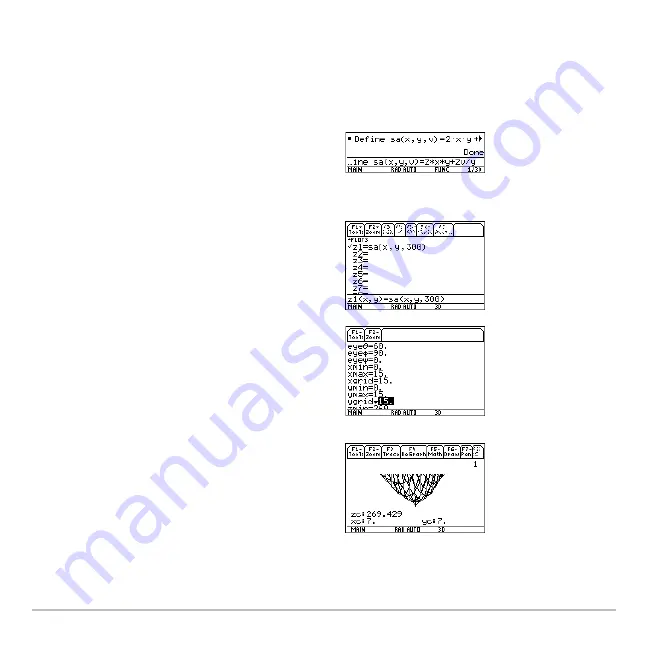
Exploring a 3D Graph of the Surface Area of a Parallelepiped
Perform the following steps to define a function for the surface area of a parallelepiped,
draw a 3D graph, and use the
Trace
tool to find a point close to the minimum surface
area.
1. On the Home screen, define the function
sa(x,y,v)
for the surface area of a
parallelepiped.
Enter:
define sa(x,y,v)=2 † x † y + 2v/x+2v/y
2. Select the 3D Graph mode. Then enter
the function for
z1(x,y)
as shown in this
example with volume
v=300
.
3. Set the Window variables to:
eye= [60,90,0]
x=
[0,15,15]
y= [0,15,15]
z= [260,300]
ncontour= [5]
4. Graph the function and use
Trace
to go to
the point close to the minimum value of
the surface area function.