Tel: 886. 909 602 109 Email: [email protected]
www.salukitec.com
10
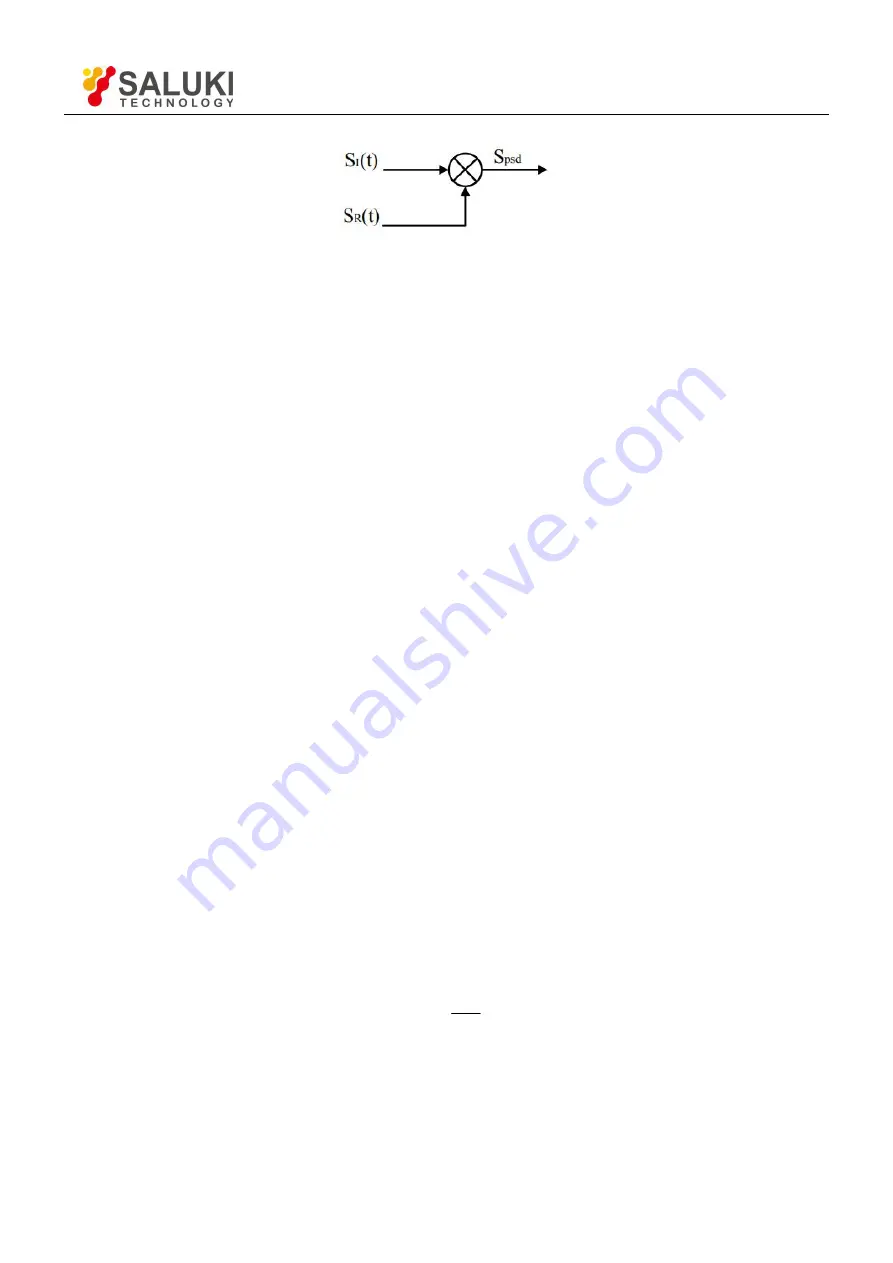
Fig.5 PSD diagram
Because the PSD based on analog method has temperature drift, there are always some deviation between the output and
actual result that is the uncertain system error. On the other hand, the PSD based on digital method has a precise amplitude
that never change, so it will not generate any system errors. This eliminates a major source of gain error found in the linear
analog lock-in. Because the inputs of analog multiplier are analog quantity, the reference signal is also affected by
temperature drift, which will cause greater errors in the results of coherent modulation.
The dynamic reserve of an analog PSD's is limited to about 60 dB, because there are always many background noises.
When there is a large noise signal present, 1000 times or 60dB greater than the full-scale signal, the analog PSD measures
the signal with an error. Because the lock-in amplifier is mainly used to detect weak signals, when the amplitude of
background noise is similar to or larger than the signal amplitude, the results of coherent modulation will be wrong.
To the digital PSD's, the dynamic reserve is limited by the quality of the A/D conversion. Once the input signal is
digitized, no further errors are introduced. Practically, the dynamic reserve of SE1022D can exceed 120dB.
The performance of a lock-in amplifier is largely determined by the performance of its PSD's. Almost in all respects, the
digital PSD outperforms the analog one. Besides, the digital PSD is also more convenient to modify.
1.5 Time Constants and DC Gain
The output signal of the PSD contains many signals of various frequency, such as the sum or difference between the input
signal frequency and the reference frequency. Only the signal whose frequency is exactly equal to the reference frequency
will result in a DC output.
The low pass filter (LPF) at the PSD output removes all the AC signals that unwanted, including the 2F (sum of the
signal and the reference) and the noise signals. This filter is what makes the lock-in such a narrow band detector.
Time Constants
The bandwidth setting of the low pass filter is the same as the conventional low pass filter. They are both determined by
the time constants. The calculation of the time constant is defined as:
f
TC
2
1
Here f is the -3dB frequency of the low-pass filter. For example, to a one-order low pass filter of RC type, a 1s time
constant means its -3dB point occurs at 0.16Hz.
In fact, where there is an input noise, there is an output noise. By increasing the time constant, the output becomes more

























